Interleaving Practice

I have learned that Interleaving Practice is an "official learning technique" from my favorite author - Barbara Oakley and I am glad she took the time and effort to educate the world about it. I would not be surprised, however, to hear from someone else, that they had been doing the same thing for a long time... The reasoning behind it makes absolute sense to me, and is very well described in the video from GoCognitive that you see in this post.
Since I teach a high school Geometry for both - regular (1-year) and a 2-year Common Core sequences, - I have to have a flexible scaffold-able unit for each of the big ideas. A unit, that would allow to teach at different learning rates a) prerequisite material, b) material that needs to be spiraled, and c) the new material. Sounds like a job for Interleaving practitioner!
I have been intentionally using this practice (or so I want to believe) in my instruction for a few years now. This holiday season I, finally, devoted some time to neatly organize my Area and Perimeter Unit.
...My next post will be an overview of the results...

High SchoolConnections to this Topic Can be by Far More Intresting than just these obvious connections...

How I Teach Making Use of Structure and Expressing Regularity in Repeated Reasoning

   Many Geometry teachers know, that students do not buy into these two Mathematical Practices right away: they are more likely to skip to conclusions (often-incorrect ones) and write down their answers (if they, actually, get to any). It takes a big effort and perseverance on both sides (tchrs' and ss') to finally get clear justifications in students' work, which are the demonstration of "Making Sense of  Problems and Persevere in Solving Them".

Approach learning in strides.

   I do not know how much of this struggle is about overcoming students' disbelief  in the need for this extra effort and how much comes from the fact that they feel overwhelmed and confused with the steps, since they do not, yet, have clear "cause-and-effect" connections within a new topic. This might be individual, but I did notice, that when they work with flow-charts, mind maps or at least solved-examples, there is less of that resentment and more of communication between my learners. Over time I came to the conclusion, that we (ss and I) were getting better results, if we approached learning in strides. I am attaching a GIF that shows the break-down of how I teach basic Geometry terms, relationships and diagramming. Feel free to download it. It the previous post you can find a video with the same GIF, in case it is easier for you to use.
    First, we want to have strong connections between just a few Geo vocabulary terms, such as Segment Bisector-Midpoint-Congruent Segments (see my earlier post about it HERE).

Practice structure and making connections

   Then, we want to show consistent applications of those terms to various problems. That is where we practice structure and make connections with similar situations or draw conclusions that there are no connections, and justify why. We work with this sort of practice using only one idea at a time (ex. Segment Bisectors; Angle Bisectors; Special Angle Pairs).
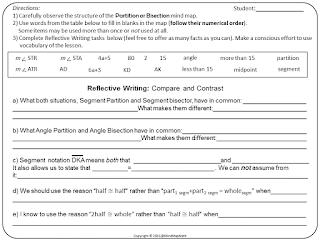
   I included the summary mind map, which lures students into such exercise and may be used either on four different occasions, or during a summary lesson.

Ask students to zero-in on certain aspects of the topic.

   Third stage usually involves interleaving practice (more on this HERE), which calls for students' focus on "comparing and contrasting" between all terms withing a unit and related situations. This is when I would normally give a follow-up writing assignment, asking students to zero-in on certain aspects of the topic. This is a sample of such assignment.

Wait, what just happened? I thought they got it yesterday?!

   We, teachers, experience it almost every week: our kids may "get" the material on a day-to-day basis,when it is taught one idea at a time, but once they are at the point when they have to deal with a mixed bag of questions, some of students have trouble even starting. I believe that it is very important to let our students know of this very well-recognized psychological phenomenon and that it should be expected.
   Every learner has experienced it at some point: as beginners, we might know chunks of information, but most likely, we have not, YET, see connections between them or even developed retrieval mechanisms for initiating them. Sometimes getting over this hump takes us longer then other times...but being aware of it, definitely helps students avoid much of the frustration related to the process.  
   It is very easy for us, teachers (aka masters of the topic at hand)  to take for granted our ability to see the whole picture, and underestimate the value (and magnitude) of students' struggle here. Meanwhile, this experience can be transformed into one of those GRAND teachable moments, that, perhaps, explain why we want our children to learn Mathematics...It shows, that mastery starts with learning facts, comparing and contrasting, deciphering through relevant traits, trying them out, and, maybe, identifying illusions of competence, and tuning up the process along the way. Mastery comes with perseverance ...just like in real life...
   INTERLEAVING -doing a mixture of different kinds of problems-is what I use to help students to move from a novice to an experienced learner of the topic. Below is a short video of  the mind map that my students will have by the end of the Units on Basics of Segment and Angle Relationships. We summarize new material into CHUNKS reflected by this map (same order as in the clip) after learning new terminology, use of symbols and appropriate diagramming. At the end of the unit, we use this map to help with learning how to shift our mental gears from one situation to another. The poster of this map became a very handy reference when we needed to bring back the structure of communicating about geometric relationships throughout the school year. 
   Usually, these two topics are taught in the beginning of a course, which makes them vital for an easy student transition to the new level of expectations to their work. I find, that students whose attendance was spotty during this time, have a much harder way of finding a good standing with the subject. I can not control their attendance, but at least I can make an attempt to help them catch up. Later on, I will be posting the videos that give a thorough overview of the material in the CHUNKS of this map.